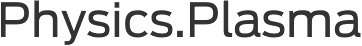Binding energy of tritium

Mass and energy are related to each other by the equation

E = energy measured in joules (J)
m = mass measured in kilograms (kg)
c = the speed of light 3 x 108 ms-1

This equation tells us that mass can be converted into energy and energy can be converted into mass.

The masses of particles are often given in atomic mass units (u) rather than in kilograms however we can convert between the two units when we need to;

1 u = 1.661 x 10-27 kg

or we could look at the energy equivalent of 1 u in mega electronvolts (MeV)

1 u = 931.3 MeV

Mass Difference (mass defect)

The mass difference of a nucleus is the difference between the total mass of the separate nucleons added together compared to the mass of the nucleus when all the nucleons are together in the nucleus.

For example if we look at the 2 protons and 2 neutrons that make up a helium nucleus separately they have a total mass of 4.031882 u we would expect the mass of the helium nucleus to be 4.031882 u as well but in fact it is only 4.001505 u. When the nucleons were combined together in the nucleus the mass of the helium nucleus was 0.030377 u less then we expected, that is the mass difference.

Binding Energy

The mass difference described above represents a loss of energy. The helium nucleus has less energy than the separate nucleons had (remember E=mc2 mass and energy are interchangeable). This is the binding energy.

The binding energy of a nucleus is the energy which would be required to separate the nucleus into its individual separate nucleons.

So for the helium example above with a mass difference of 0.030377 u the binding energy is;

0.030377 x 931.3 = 28.3 MeV

Binding Energy per Nucleon

If you divide the binding energy by the number of nucleons you have the binding energy per nucleon, so for the helium example above;

binding energy per nucleon = binding energy / number of nucleons

binding energy per nucleon = 28.3 / 4 = 7.1 MeV per nucleon

Graph of average binding energy per nucleon against nucleon number

The greater the amount of binding energy per nucleon the more stable a nucleus is, iron has the greatest amount of binding energy per nucleon.

This graph suggest two ways to get energy out of nuclear reactions (i.e. to increase the binding energy per nucleon);

• Nuclear Fission – taking heavy nuclei (like uranium-235) and splitting them up into lighter nuclei (like barium & krypton).
• Nuclear Fusion – taking lighter nuclei (like deuterium and tritium, isotopes of hydrogen) and fusing them into heavier nuclei (like helium).

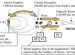
Nuclear Fission

When a neutron hits a uranium nucleus and is captured it causes the nucleus to split into two lighter nuclei and two further neutrons are released. Energy is released in this process mainly in the form of kinetic energy of the fission products.